Aperiodic Monotiles
June 22, 2023
No Comments
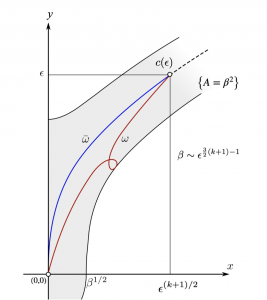
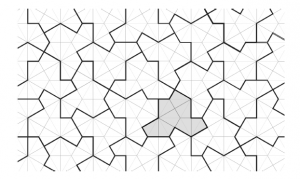
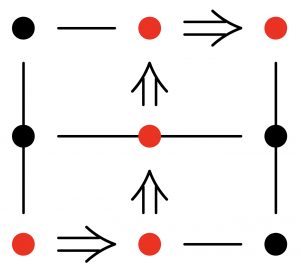
Author: Marjorie Senechal. “A longstanding open problem asks for . . . a shape that admits tilings of the plane, but never periodic tilings. We answer this problem for topological disk tiles by exhibiting a continuum of combinatorially equivalent aperiodic polygons.”
Thus begins the abstract of the preprint “An Aperiodic Monotile,” first posted on arxiv.org on March 20, 2023 by David Smith, Joseph Myers, Craig Kaplan, and Chaim Goodman-Strauss ([1]). Propelled by social media, lively international Zoom gatherings, on-line science magazines, and The New York Times, the news streaked around the globe at (nearly) the speed of light.
